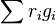Augmentation ideal
In algebra, an augmentation ideal is an ideal that can be defined in any group ring. If G is a group and R a commutative ring, there is a ring homomorphism  , called the augmentation map, from the group ring
, called the augmentation map, from the group ring
to R, defined by taking a sum
to
Here ri is an element of R and gi an element of G. The sums are finite, by definition of the group ring. In less formal terms,
is defined as 1R whatever the element g in G, and  is then extended to a homomorphism of R-modules in the obvious way. The augmentation ideal is the kernel of
is then extended to a homomorphism of R-modules in the obvious way. The augmentation ideal is the kernel of  , and is therefore a two-sided ideal in R[G]. It is generated by the differences
, and is therefore a two-sided ideal in R[G]. It is generated by the differences
of group elements.
Furthermore it is also generated by
which is a basis for the augmentation ideal as a free R module.
For R and G as above, the group ring R[G] is an example of an augmented R-algebra. Such an algebra comes equipped with a ring homomorphism to R. The kernel of this homomorphism is the augmentation ideal of the algebra.
Another class of examples of augmentation ideal can be the kernel of the counit  of any Hopf algebra.
of any Hopf algebra.
The augmentation ideal plays a basic role in group cohomology, amongst other applications.
References
- D. L. Johnson (1990). Presentations of groups. London Mathematical Society Student Texts 15. Cambridge University Press. pp. 149–150. ISBN 0-521-37203-8.
- Dummit and Foote, Abstract Algebra
![R[G]](../I/m/ae1a6038ecd0b0c3de71ddc95c04c87f.png)